Porous Media

Most materials containing pores in them are considered as porous media and porous media widely exist in the world. Basically, a porous medium consists of pore portion and solid matrix or skeletal portion, The porous media in nature are usually filled with liquid or gas. Strictly speaking, most of solid materials in nature are porous media. Fig.1 shows the three main types of porous media, their typical microscopic arrangements and some of the physical models used to describe their mechanisms.

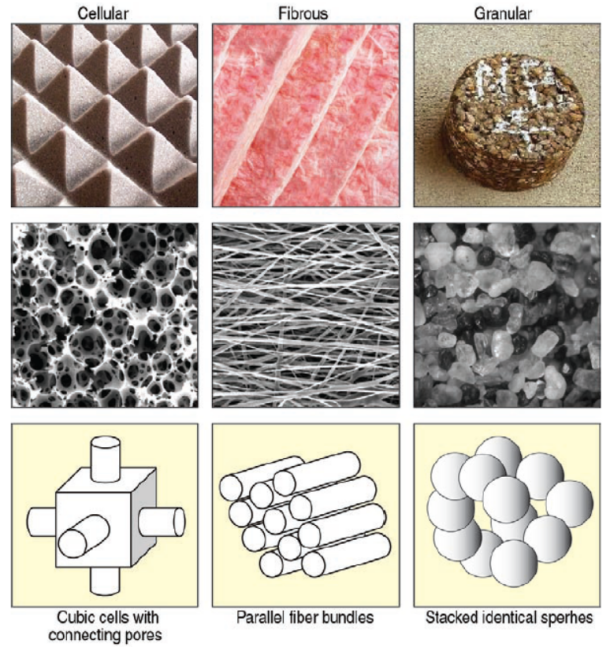
Fig.1. The three main types of porous media (Source: Ref. 2)

With the significant development of the research and applications on porous media in recent years, the concept of porous media can be seen in applying in many areas of applied science and engineering. Wave propagations in porous media have great application significances in the areas of geophysics, earthquake, civil engineering, mechanical engineering, and petroleum engineering.

Continuum Hypothesis and Fractal Theories

Due to the fact that the real structure of porous media are very complex, it is impossible to give an exact picture about the phenomenon involving the interaction of fluid and solid surface. To overcome this difficulty, one can treat the porous media as a continuum consisting of series of particles, which is very similar to the definition of fluid density. In other words, people can find a representative volume element of porous media that the size of this volume id required to be much lager than that of a single pore, which indicates that a porous medium contains many pores, which are much less than the characteristic length of the whole flow field. The porous media can then be treated as a continuum consisting of series of such elements.

For certain media, if the porosity is irrelevant to the spatial position, the media are called homogenous media. Otherwise, it is called inhomogeneous media.

The word “fractal” was first introduce by a mathematician, Mandelbrot. The parameter for quantitative description of the property of fractal is called fractal dimension. Contrary to the classical Euclid geometry, whose dimension can only be assigned as an integer because it deals with regular geometrical figures, the dimension of fractal structure is a fraction. The fractal theory is applied to potential regulations from the superficial irregularity and has been widely used among many subjects.

The main characteristics of fractal geometry are self-similar and scale invariant which means part of geometrical shape can be exactly or very close to a reduced size copy of the whole. Actually, it has been reported that the microstructure and the distribution of pore size possess the characteristics of fractal. Sierpinski triangle and Menger sponge, as shown in Fig. 2 and Fig. 3, are typical models usually used in mathematical representing the geometries of porous media.

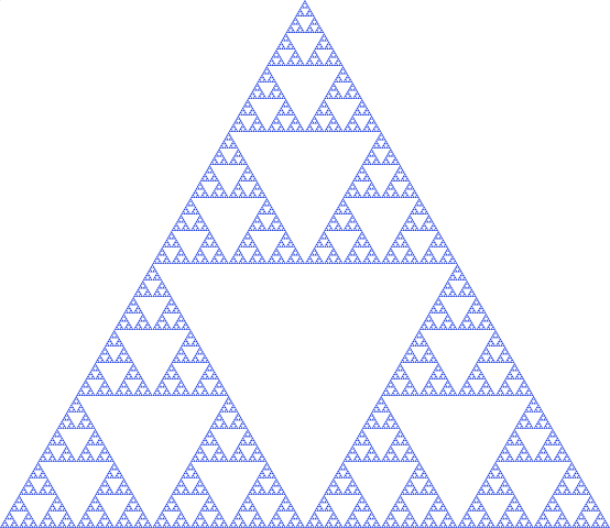
Fig. 2. Sierpinski triangle (Source: Wikipedia)

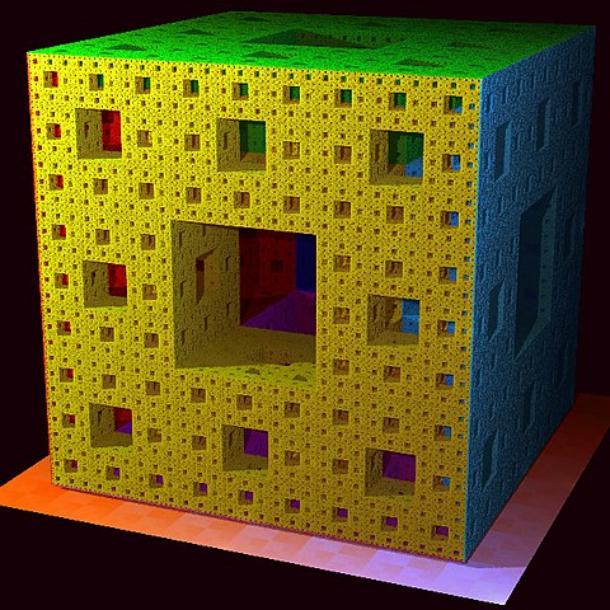
Fig. 3. Menger sponge (Source: Wikipedia)

Since the invention of fractal theory, it has been applied to investigate the transportation problem in porous media. The porosity and permeability of porous media may be related to the fractal index.

Wave Propagations in Porous Media (full space domain)

In investigating wave motions in porous media, the main concern are both the waves regarding the media in which the waves propagate and energy source by which the waves are generated. In fact, the wave motions are affected by a variety of factors such as topologic and material properties of media, characteristics of wave energy sources, medium porosity and permeability, properties of the fluids saturated in the media, as well as combinations of the waves propagation in the domain considered. The behaviors of the wave motions are therefore very complex.

For the media, the wave behavior in a fluid-saturated medium is affected not only by the separate motions of the solid and fluid but also by the relative motion between the solid and fluid. It is generally accepted that the waves attenuate while they propagate in the medium due to the material properties and the presence of the pore fluid in the porous media. In fact, wave velocity and wave attenuation are two key aspects of wave propagation in porous media. These aspects are important in analyzing the dynamic response of the media with respect to the properties of both the media and the wave source, such as viscosity, porosity, and frequency.

For the energy source, the wave propagation described in the governing equations relies on the characteristics of the energy source by which the wave is generated. Therefore, the model describing the wave field with the displacements and relative displacements between the solid and fluid for each point in the porous media domain considered is necessary.

Component of the body waves

• P-wave (pressure or compressional wave)
• SV wave (shear vertical wave)
• SH wave (shear horizonal wave)

Biot theory

Biot theory describes the elastic waves propagation in a porous saturated medium. Biot ignores the microscopic level and assumes that continuum mechanics can be applied to measurable macroscopic quantities. He postulates the Lagrangian and use Hamilton principle to derive the equations that govern the wave propagation.

The main assumptions of the theory are:

1. Infinitesimal transformations occur between the reference and current states of deformation. Displacements, strains and particle velocities are small. Consequently, the Eulerian and Lagrangian formulations coincide up to the first-order. The constitutive equations, dissipation forces, and kinetic momenta are linear. (The strain energy, dissipation potential and kinetic energy are quadratic forms in the field variables.)

2. The principles of continuum mechanics can be applied to measurable macroscopic values. The macroscopic quantities used in Biot’s theory are volume averages of the corresponding microscopic quantities of the constituents.

3. The wavelength is large compared with the dimensions of a macroscopic elementary volume. This volume has well defined properties, such as porosity, permeability and elastic moduli, which are representative of the medium. Scattering effects are thus neglected.

4. The conditions are isothermal.

5. The stress distribution in the fluid is hydrostatic. (It may be not completely hydrostatic, since the fluid is viscous.)

6. The liquid phase is continuous. The matrix consists of the solid phase and disconnected pores, which do not contribute to the porosity.

7. In most cases, the material of the frame is isotropic. Anisotropy is due to a preferential alignment of the pores (or cracks).

Equations of fluid dyanamics

Acoustic waves are small propagating fluctuations in pressure, particle velocity, mass density, entropy and temperature on lager and more uniform background values of these quantities. Let $p_0,\bm{v}_0,\rho{}_0, s_0, T_0$ refer to the pressure, partical velocity, mass density, specific entropy density (i.e., entropy per unit mass), and absolute temperature of the background medium, respectively. Then, the total pressure $P$ , particle velocity $\bm{V}$ , mass density $\varGamma{}$ , specific entropy density $S$ , and absolute temperature $T$ associated with a propagating acoustic disturbance are assumed to be

$\begin{array}{rcl} \left\{ P,\bm{V},\varGamma,S,T \right\} & = & \left\{ p_0,\bm{v}_0,\rho{}_0,s_0,T_0 \right\} \\ & + & \left\{ p,\bm{v},\rho,s,\theta \right\} \end{array}$

Similarly, the total sound speed of the medium is given by

$C=c_0+c$

Continuity Equation

The local form (i.e., the Eulerian specification of the flow field) of the principle of conservation of mass is expressed by the continuity equation (without mass source):

$\frac{D\varGamma}{Dt}=-\varGamma{}\nabla{}\cdot\boldsymbol{V}$

Where the Material Derivative $\frac{D[\bullet]}{Dt}=\frac{\partial{}[\bullet]}{\partial{}t}+\bm{V}\cdot\left(\nabla{}[\bullet]\right)$

Movement Equation

Movement Equation (or the Equation of momentum) can be derived from the Newton’s Second Law (without body force):

$\varGamma\frac{D\bm{V}}{Dt}=\nabla{}\mathbb{T}$

Here $\mathbb{T}=\sigma{}_{ij}$ denotes the Cauchy stress tensor - a second-order symmetric tensor consisted by 6 independent scalar variables.

Constitutive Equation

The Constitutive Equation explicitly relate the Cauchy stress tensor $\sigma{}_{ij}$, the normal stress vector components $P_i$ and the velocity vector component $U_i$. For isotropic Newtonian fluid, with the Volume viscosity introduced by Stokes in 1949:

$\begin{cases} \sigma{}_{ij}=-P\delta{}_{ij}+T_{ij} \\ T_{ij}=\mu{}'\theta{}\delta{}_{ij}+2\mu{}d_{ij} \\ \theta{}=d_{11}+d_{22}+d_{33} \\ d_{ij}=\frac{1}{2}\left[ \frac{\partial{}U_i}{\partial{}x_j}+\frac{\partial{}U_j}{\partial{}x_i}\right] \end{cases}$

Where $\mu$ is the shear viscosity, and $\mu{}'$ is the volume viscosity of the medium. Here we considered the viscous effect of the medium.

Equations of State

1. nonlinear effect

$\left( \frac{\partial{}^2P}{\partial{}\varGamma{}^2} \right)_{S,\rho{}_0}=2c_0^2\frac{\beta{}-1}{\rho{}_0}$

Here $\beta$ is the nonlinear coefficient.

2. heat conduction effect

$\begin{array}{rcl} P & = & p_0+c_0^2\rho{}+\frac{1}{2}\left(\frac{\partial{}^2P}{\partial{}\varGamma{}^2}\right)_{S,\rho{}_0}\rho{}^2 \\ & - & \kappa\left(\frac{1}{C_v}-\frac{1}{C_p}\right)\nabla{}\cdot{}\bm{V} \end{array}$

Here $\kappa{}$ is the thermal conductivity of the medium.

3. relaxation effect

$\begin{array}{rcl} P & = & p_0+c_{\infty}^2\rho{}+\frac{1}{2}\left(\frac{\partial{}^2P}{\partial{}\varGamma{}^2}\right)_{S,\rho{}_0,\xi{}_0}\rho{}^2\\ & - & \frac{mc_0^2}{\tau{}_r}\int_{-\infty}^t\rho{}e^{-\left(t-t'\right)/\tau{}_r}dt' \end{array}$

Here, $\tau{}_r$ is the relaxation time, $c_\infty$ is the sound speed when $\omega{}\tau{}_r \to \infty$, $m$ is a dimensionless parameter which describing the dispersion and dissipation characteristics of the medium.

Linearization of the control equations

It is well know that the Helmholtz Equation is derived from the nonlinear acoustic theories by serial hypothesis. The viscous effect, nonlinear effect, heatconduction effect and relaxation effect can be neglected under these assumptions.

• the medium is ideal fluid.
• acoustic waves is much smaller disturbance then background medium.
• frequency of the acoustic wave is large enough that the propagation of the sound can be regard as an adiabatic process.
• the background medium is uniformly and stationary.

How to discribe those effects

I will discuss when should we apply linear acoustic theory instead of the nonlinear one based on 1-D control equations.

Mach Number

Mach Number is a dimensionless quantity representing the ratio of flow velocity to the local speed of sound.

$\bm{Ma}=\frac{U}{C}=\frac{u_0+u}{c_0+c}$

Where, $U$ is the component of total particle velocity $\bm{V}=U_i$

Acoustic Reynolds Number

In fluid dynamics, the Reynolds Number is a dimensionless quantity representing the ratio of inertia force to viscous force.

$\mathcal{Re}=\frac{\Gamma{}\bm{V}\cdot{}\nabla{}\bm{V}}{\mu{}\nabla{}^2\bm{V}}\approx\frac{\varGamma{}UL}{\mu}$

Here, $L$ is a characteristic linear dimension. Similarly, we compare the convection term with the dissipative term of the Burgers Equation and got it’s acoustic Reynolds Number. Bugers Equation (no rotation) is derived from the continuity equation, movement equation and the equation of state which considered nonlinear effect and heat conduction effect.

$\begin{array}{rcl}\varGamma\frac{DU}{Dt}&=&\varGamma{}\frac{\partial{}U}{\partial{}t}+\varGamma{}U\frac{\partial{}U}{\partial{}x}\\&=&-c_0^2\frac{\partial{}\varGamma{}}{\partial{}x}-c_0^2\frac{\beta{}-1}{\rho_0}\frac{\partial{}\rho{}^2}{\partial{}x}+b\frac{\partial{}^2U}{\partial{}x^2} \end{array}$

Here, $b=\mu{}'+2\mu{}+\kappa{}\left(\frac{1}{C_v}-\frac{1}{C_p}\right)$ . With the monochromatic traveing wave solution, the acoustic Reynolds Number can be derived

$\bm{Re}=\frac{\varGamma{}U\frac{\partial{}U}{\partial{}x}}{b\frac{\partial{}^2U}{\partial{}x^2}}\sim{}\left|\frac{\frac{U}{c_0}\omega{}\rho_0{}U}{b\omega{}^2U}\right|=\frac{U\rho_0c_0}{b\omega}$

When the backgound medium is stationary, the acoustic Reynolds Number can be write as

$\bm{Re}=\frac{u\rho_0c_0}{b\omega}$

When to use

1. $\bm{Ma}<0.3$ , that means the total particle velocity is much smaller than sound velocity, the background medium is uncompressable (i.e., the mass density is uniform) and nearly stationary.
2. $\mathcal{Re}\gg{}1$ , that means the $\mu$ is neglectable small, the medium can be considered as an ideal fluid (i.e., the viscous effect can be neglect).
3. $\bm{Re}\gg{}1$ , that means the nonlinear effect and heat conduction effect can be neglect (i.e., acoustic waves is much smaller disturbance then background medium and the propagation of the sound can be regard as an adiabatic process).

If all 3 conditions are met and we can apply linear acoustic theories?

No, the discussion of the relaxiation effect is needed.

---

Reference

[1] Campos, L. M. “On 36 forms of the acoustic wave equation in potential flows and inhomogeneous media.” Applied Mechanics Reviews 60.4 (2007): 149-171.

[2] Qian, zu . Fei Xian Xing Sheng Xue. Bei jing: Ke xue chu ban she, 2009. Print.

[3] Solui︠a︡n, Stepan Ivanovich. Theoretical foundations of nonlinear acoustics. Consultants Bureau, 1977.

Welcome to NiSiC! This is an individual blog powered by Hexo, hosted on Github.

I’m the website holder - an undergraduate student who is major in Marine Engineering at Harbin Engineering University. I’ll post some excerpts or reviews of the fields what am I interested in. The site is serving as an academic information memo of mine.

Thanks for visiting 😉